Theorem 1.
Let the function f (x) be continuous at x = a and let C be a constant. Then the function Cf (x) is also continuous at x = a.
Theorem 2.
Let the functions f (x) and g (x) be continuous at x = a. Then the sum of the functions
f (x) + g (x) is also continuous at x = a.
Theorem 3.
Let the functions f (x) and g (x) be continuous at x = a. Then the product of the functions f (x) g (x) is also continuous at x = a.
Theorem 4.
Let the functions f (x) and g (x) be continuous at x = a. Then the quotient of the functions
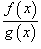is also continuous at x = a assuming that
.
