Short theoretical information about estimation of uncertainty of measurement results of physical quantities.
по лабораторной работе №28
“Измерение удельного заряда электрона методом магнетрона”
Студент: Леонов Александр Группа: Р-122 КТ Дата: 23.10.01
г.Краснотурьинск 2001г. I. Расчетная формула для определения удельного заряда электрона с пояснениями смысла величин, входящих в нее.
где Ua=5,25 В – разность потенциалов между катодом и анодом, L – длинна соленоида, D – диаметр соленоида, Ra – радиус анода, μ0= 4* 10-7Гн/м – магнитная постоянная, Ic,kp – критический ток в соленоиде, N – число витков соленоида.
II. Средства измерений и их характеристики.
Магнетрон: А) Соленоид – диаметр D=80 мм, длинна L=120 мм, число витков N=200; ΔD=1мм, ΔL=1мм; Б) Диод – радиус анодаRa=3,36мм, ΔR=0,01мм. Погрешности: А) ΔUa= θ; осн.=в (задается в таблице к установке); Б) ΔIc,кр=А (задается в таблице к установке).
III. Схема электрической цепи.
IV. Результаты измерений (в форме табл. 1).
Таблица 1 Зависимость анодного тока от тока в соленоиде
V. Построение графика Ia=f(Ic).
VI. Определение критического тока Ic, кр в соленоиде по графику, построенному по данным табл. 2.(13
Таблица 2
VII. Удельный заряд электрона
VIII. Оценка границ погрешностей результата измерения:
IX. Окончательный результат |e|/m=(6,8868 ±0,0007)*1010 Кл/кг
X. Выводы: В результате проделанной работы я познакомился с измерением заряда электрона методом магнетрона, получил зависимость анодного тока от тока в соленоиде. В результате получил конкретные значения, которые сравнил с теоретическими. Между ними появились расхождения, т.к. погрешность имеют все приборы (особенно большую погрешность имеет вольтметр), в проводниках имеется активное сопротивление, диаметр и длинна соленоида рассчитаны не точно и многое другое, а так же не совершенность метода. По графику прослеживается зависимость анодного тока от тока в соленоиде. Short theoretical information about estimation of uncertainty of measurement results of physical quantities.
Physical quantity - is defined as property which is common in a qualitative sense for many physical objects (physical systems, their states and processes occurring in them), but in a quantitative sense individual for each object. The size of physical quantity is named as quantitative content of properties in this object, corresponding to the notion of a physical quantity. Unit of physical quantity - physical quantity of a certain size, adopted in agreement for the quantitative mapping of homogeneous with it values. Various units of the same size vary in size. The size of units of physical quantities - quantitative characterization of the unit defined by the number of values in the unit of measurement. The size of basic units is established arbitrarily and independently of one another by definitions. The size of the derivative unit is determined by the type of depence between the quantities and sizes of units and the established from equations which define this unit from the basic or other derived units. The dimension of the of PV - an expression showing connection of the value of physical quantities are the basis in system of units. The dimension is the qualitative characteristic of FV can be written as a product of symbol (dimensions) corresponding to the basic values, raised to some degrees, called the dimension indices. The numerical value of the dimension indices determined from the connection equation of derivatives and the main physiacl values. Values, in which all the main values are zero degree, called dimensionless. Measurements, as experimental processes are varied.This is explained by many experimental quantities the different character of the measurement values, the different requirements of accuracy, etc. The purpose of measurement is to determine the value of the measured quantity, i.e. values of a certain size, which must be measured. Therefore, the measurement starts the corresponding specification of the measured value, the measurement method and measurement procedure. Most widespread classification of the types of measurements, depending on the method of processing the experimental data. In accordance with the classification of the measurement can be divided into direct, indirect, joint and cumulative. Direct measurement - a measurement in which the required value of a physical quantity is directly from experimental data by comparing the measured value with the standards (measurement of the length by ruler, measure the voltage by voltmeter). Indirect measurement - measurement, in which the required value of is based on the known dependence between this value and the values he chooses to direct measurements (for example, we find the resistance of resistor on the basis of Ohm's law by substituting the current and voltage values obtained by direct measurements.) Joint dimension - the simultaneous measurement of several variables which required values variables are solution of the system consisting of a result of direct measurements of various combinations of these variables. The combined measurement - simultaneous measurement of multiple variables of the same name, in which the required values of variables are solution of the system consisting of a result of direct measurements of various combinations of these variables. Currently, evaluation of the quality of PV measurement performed using the term "measurement uncertainty". The word "uncertainty" means doubt and, thus, in its broadest sense, the "measurement uncertainty" means doubt about the reliability of the measurement result. In the narrow sense of the uncertainty of measurement - is a parameter related to the measurement result, which characterizes the scattering of the values that could reasonably be assigned to the measurand. In other words, the uncertainty of measurement is understood as incomplete knowledge about the importance of the measured value, and to quantify the incompleteness of the use of the probability distribution of possible law (reasonably assigned) values of the measured value. The parameter of this distribution (also called - uncertainty) quantifies the accuracy of the measurement result. The conceptual basis of the replacement of the term "error" in the measurement result, the term "uncertainty" is the philosophical premise of agnosticism about what the true meaning unknowable, and the error based on the use of the true value of the measured value, looses meaning. In the classical approach to the estimation the accuracy of measurements was taken as a postulate that: a) There is the true value of the measurand;
In the current international and Ukrainian regulations [ 1, 2, 3 ] provides a new concept of processing the results of measurements of PV. The main thing in it is:
where x1,..., xm - input values (or other directly measured quantities influencing on the measurement); - calculation by type B - using other methods. Calculation of uncertainty according to Type A Initial data for calculation of HA by type A is a statistical number of measured input variables: {xq} (where q = 1,..., m).
Where The standard uncertainty of HA (xq) measurement range of the input variable {xq} (where q = 1,..., m) at which the result is determined as the arithmetic mean is calculated by the formula:
The total standard uncertainty (HcA) in the case of uncorrelated estimates of indirect measurements of ejection fraction ((x 1,…, хm) is defined as:
Where In the case of direct measurements of the total standard uncertainty is given by:
In evaluating the uncertainty of the result of indirect measurements of PV using the concept of correlation and covariance of random variables.
Where The correlation coefficient, which is a measure of the relative mutual dependence of two random variables is equal to the ratio of their covariance to the positive square root of the product of their variances.
Where і, j = 1, 2, …, n; k, q = 1, 2, …, m. To calculate the correlation coefficient r(xk, xq) using a matched pair of measurements (xkd, xqd) (where d = 1,..., nkq; nkq --the number of matched pairs of measurements). Calculation of uncertainty of type B The initial data used to calculate HB: To calculate the standard uncertainty of type B - H B (xq), influencing factors should be considered: 1) The technical characteristics of the device (the limit of the basic relative error limit of the additional error of the device, the resistive component of the input resistance of the device),
Where l = 1, 2, 3 …. L, a – the main error of the voltmeter, ξ – factors influencing on the measurement result. The total standard uncertainty of voltage measurement result is as follows:
Where
Standard uncertainty caused by the basic error of the voltmeter, calculated according to the type B — H аB (U), determined by the formulas:
where a - the main error of the SIT in terms of the accuracy class of instrument. For other distribution functions used by other forms provided in Appendix A.
where UV – the voltmeter results; Moreover, it is necessary to take into account uncertainty in the input impedance H R вх uncertainty of load resistance H R НAlso it is necessary to determine the correction (ρ;):
Measurement uncertainty introduced by the amendment (ρ) is given by:
Where the sensitivity coefficients
To estimate the uncertainty НТ0 В, due to deviation from the normal temperature Тнорм = 20˚С assumed that the limit of the instrument additional error caused by deviation of the ambient temperature (Токр.ср). within the normal operating temperature of the area, which will not exceed the limit of basic error for every 10 ˚ C temperature change is:
For digital equipment necessary to calculate the uncertainty of the quantization Нкв. В (U), defined by the boundaries of the quantization error (
In the above example suggests that a uniform distribution of the probability of errors in the SIT, the parameters of the object. The total standard uncertainty Нcв with indirect measurements in the case of uncorrelated estimates x1,…, хm is calculated by formula:
where The resulting standard uncertainty is determined by the formula:
Expanded uncertainty of Hp given by multiplying the resulting standard uncertainty of measurement result in the coverafe factor (k).
In general, the coverage factor k is selected in accordance with the expression
where The freedom degree - a characteristic of PV. The number of degrees of freedom determines the minimum number of independent variables required for a complete description of the process under investigation. Also, the number of degrees of freedom equal to the total number of independent numbers.
where For direct multiple measurements n eff = n – 1 In many practical cases, when calculating the uncertainty of measurement results and speculate about a normal distribution of possible values of the measured value and assume: k = 2 if р ≈ 0,95 and k = 3 if р ≈ 0,99. With the assumption of uniformity of the distribution law considered: k = 1,65 if р ≈ 0,95 and k = 1,71 if р ≈ 0,99. When you write the measurement result is the actual value of the measured value (y), the resulting total uncertainty (Н∑(y)), expanded uncertainty (Hp), the effective number of freedom degrees ( Laboratory work №8 Estimation of uncertainty of measurement results of physical values Part 1. Estimation of uncertainty of the results of direct measurements of the voltage of information signals. 1. Purpose of work 1.1. To study the basic kinds and types of uncertainty of measurement results of physical quantities, a methodology for assessing the uncertainty of type A and type B. 2. Key positions 2.1 Uncertainty of measurement - parameter related to the results of measurements and characterizes the scattering of the values that could reasonably be assigned to the measurand. 2.3 Calculation of uncertainties of type A. The initial data for calculation of H А were measured by a number of statistical input variable: xq. After that it is necessary to calculate standard uncertainty of single measurement of q-th input variable H Aq (2) 2.4 During the estimation of uncertainty of measurement results of type B using such formulas (6), (7), (8), (9), (10), (11). The standard uncertainty of H B (a, ξ) is determined by the formulas for the distribution functions (rectangular, trapezoidal, triangular), the main and additional errors (Appendix A), where a - is determined by the accuracy class instrument. 2.5 The resulting standard uncertainty is determined by the formula (14). 2.6 The effective number of degrees of freedom is determined by - n eff = n - 1. 2.7 Expanded uncertainty (H p) obtained by multiplying the resulting standard uncertainty of measurement results on the enrollment ratio (k) (15).
|



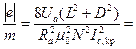 6,8868772*1010Кл/кг
6,8868772*1010Кл/кг
 ,
,
 - average arithmetic of measurements q-th input variable; n - number of measurements of the input variable.
- average arithmetic of measurements q-th input variable; n - number of measurements of the input variable. .
.
 .
.
 - sensitivity coefficient.
- sensitivity coefficient. .
.
 ,
,
 - correlation coefficient
- correlation coefficient ,
,
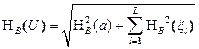 ,
,
 ,
,
 - uncertainty caused by the basic error of the voltmeter;
- uncertainty caused by the basic error of the voltmeter; - uncertainty caused by temperature changes;
- uncertainty caused by temperature changes; - standard uncertainty caused by shunting the output impedance of the object input resistance of the SIT.
- standard uncertainty caused by shunting the output impedance of the object input resistance of the SIT.
 ,
,
 – voltage on the load.
– voltage on the load. ,
,
 ,
,
 ,
,  ;
; – uncertainty of the load resistance and H R вх – uncertainty in the input resistance. Since the value of load resistance and input impedance are known about and is, within certain limits [ R min; R max], [ R вхmin; R вхmax], considering all the values of resistors within the marked boundaries equally, we estimate their uncertainty as:
– uncertainty of the load resistance and H R вх – uncertainty in the input resistance. Since the value of load resistance and input impedance are known about and is, within certain limits [ R min; R max], [ R вхmin; R вхmax], considering all the values of resistors within the marked boundaries equally, we estimate their uncertainty as:

 .
.
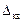 ) taking into account a uniform distribution law:
) taking into account a uniform distribution law: .
.
 ,
,
 - standard uncertainty of measurement results of q-th input value.
- standard uncertainty of measurement results of q-th input value.
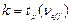
 - quantile of the Student distribution with effective degrees of freedom veff and the confidence probability (confidence level), p. The values of the coefficient
- quantile of the Student distribution with effective degrees of freedom veff and the confidence probability (confidence level), p. The values of the coefficient 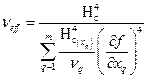 ,
,
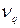 - number of degrees of freedom during the estimation of q-th input value;
- number of degrees of freedom during the estimation of q-th input value; 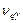 ) and confidence level (р д).
) and confidence level (р д).