The derivative of the function. Geometric and mechanical meaning. Table of derivatives. The differential of a function
Theoretical questions: 1. Definition of derivative. 2. The geometric meaning of derivative. 3. The basic rules of finding derivatives. 4. Derivatives of the basic elementary functions.5. Derivative of composite functions, implicit functions, the functions specified parametrically, the method of logarithmic differentiation. 6. Application of the differential to approximate calculations. Classroom assignments: 1. Find derivatives of following functions: 1) 4) 7) 11) 2. Calculate derivatives of following functions defined implicitly: 1. 3. 3. Calculate derivatives of following functions defined parametrically: 1. 4. U sing the method of logarithmic differentiation calculate derivatives of following functions: 1. 4. 5. Find differential of functions: 1. 4. 6. Find the approximate value: 1.
Homework: Theoretical material:L'Hopital's rule. Investigation of the function. Extremum of the function. Convexity, concavity and inflection points. Asymptote. Solve problems: 1. Find derivatives of the following functions applying the formulas and rules of differentiation: а) в) д) 2. Calculate derivatives of functions: 1. 4. 3. Show that the function 4. Find differentials of functions: а) 5. Using a differential calculate approximately: а) 6. For function
|

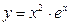 ; 2)
; 2) 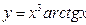 ; 3)
; 3) 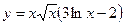 ;
;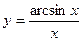 ; 5)
; 5) 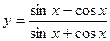 ; 6)
; 6)  ;
;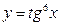 ; 8)
; 8) 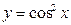 ; 9)
; 9) 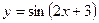 ; 10)
; 10) 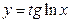 ;
;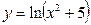 ; 12)
; 12) 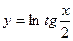 ; 13)
; 13)  .
.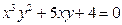 2.
2. 
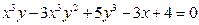 4.
4. 
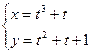 2.
2.  3.
3. 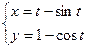 4.
4. 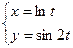
 2.
2.  3.
3. 
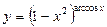 5.
5.  6.
6. 
 2.
2. 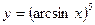 3.
3. 
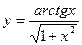 5.
5. 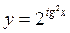
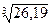 2.
2. 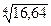 3.
3. 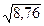 4.
4. 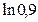 5.
5. 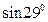 6.
6. 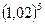 7.
7. 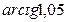
 ; б)
; б) 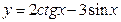 ;
;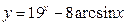 ; г)
; г) 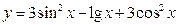 ;
;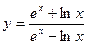 ; е)
; е) 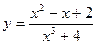 .
.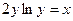 2.
2. 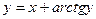 3.
3. 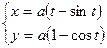
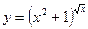 5.
5. 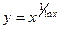
 satisfies the relation
satisfies the relation 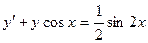 .
. ; б)
; б)  в)
в) 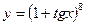
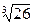 ; б)
; б) 
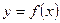 а)
а) 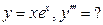 б)
б)