I.e. the intensity of the distributed load is equal to the derivative of the shearing force with respect to the bar section abscissa
Fig. 5.7.
Let us determine the bending moment at the section with the abscissa z by taking the sum of the moments of the forces located to the left from the section. For it replace the distributed load by its resultant on the region of the z length which is equal to By analogy determine the bending moment at the next section located from the left support on the distance z+dz:
Subtracting the bending moment at two sections we get the increment of the bending moment
The expression in the parentheses is the shearing force
from where
I.e. the shearing force is equal to the derivative of the bending moment with respect to the bar section abscissa. Taking the derivative of both parts of the equality (5.4), we get
|


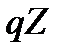 as applied in the region middle at a distance
as applied in the region middle at a distance  to the section
to the section 
 (5.2)
(5.2)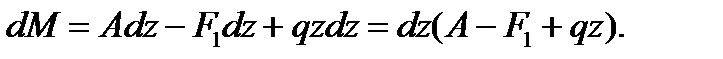 (5.3)
(5.3) . Consequently
. Consequently (5.4)
(5.4)
 (5.5)
(5.5)


